Scientific Visualization in Astronomy: The Solar Journey
project was undertaken with NASA support (NAG5-8163, NAG5-11999) in
collaboration with the University of Chicago beginning in 1999.
This has led to a number of projects
in virtual astronomy. See the
Project Data site
for examples of packages
supported under this program, including the Solar Journey package.
(Note that the AISRP Code and Algorithm Library that used to
be at " https://aisrp.nasa.gov/cal/SessionDriver/Packages/ "
has been abandoned and decomissioned by NASA for some unknown reason.)
An example of our work on star rendering embedded in the
Solar Journey package may be
found in this QuickTime movie depicting simulated
stars, which compares favorably
to real images such as Akira Fujii's
Orion.
Selected papers of ours in this area:
|
Visualizing Multiwavelength Astrophysical Data,
Hongwei Li, Chi-Wing Fu, and Andrew J. Hanson,
TVCG, Nov/Dec 2008, 14, no. 6, pp. 1555-1562,
Proceedings of IEEE Visualization 2008. Describes
a unique interactive GPU-driven volume-rendering
paradigm tailored to the study of all-sky multispectral astrophysical
data.
Paper web site.
|
|
Visualizing Large-Scale Uncertainty in Astrophysical Data,
Hongwei Li, Chi-Wing Fu, Yinggang Li, and Andrew J. Hanson,
TVCG, Nov/Dec 2007, 13, no. 6, pp. 1540-1647;
Proceedings of IEEE Visualization 2007. Astrophysical data
is characterized by a wide variety of uncertainties and error sources;
this work provides a set of tools for examining and visualizing these
features.
Paper
and web site.
|
|
Scalable WIM: Effective Exploration in Large-scale Astrophysical
Environments, TVCG, Sept/Oct 2006, 12, pp. 1005-1011;
Proceedings of IEEE Visualization 2006.
Describes a World-in-Miniature interface design for astrophysical
exploration whose development was led by Yinggang Li in my laboratory.
Paper and web
site. |
|
A Transparently Scalable Visualization Architecture for Exploring
the Universe, TVCG, Jan/Feb 2007, is a full
description of work done mainly by Chi-Wing Fu in my laboratory. This
framework supports
transparent interactive navigation across enormous scale ranges
such as those naturally occurring in astronomy.
Paper and web site. |
Architectures for Very Large Scale Visualization of Astrophysical Environments.
Our initial work on handling very large scales of spacetime
in interactive virtual reality environments is described in our paper, Very large
scale visualization methods for astrophysical data, which appears in
Proceedings of Joint Eurographics-IEEE TVCG Symposium on Visualization,
May 29-31, 2000, Amsterdam, the Netherlands. This paper is part of these
published proceedings: Springer-Verlag.
Our most extensive work, which appeared in TVCG in 2007, describes the
maturation of the scaling framework developed in Philip Chi-Wing Fu's
PhD thesis, and is entitled A Transparently Scalable
Visualization Architecture for Exploring the Universe.
For details, see the journal article
DOI:10.1109/TVCG.2007.2, TVCG, January/February
2007, pp. 108-121, vol. 13. More details are available on
Philip Fu's "powers-of-10" web site.
A local copy can be found
here.
|

|
Approach to the Black Hole at the Galactic Core.
This animation represents an assembly of data collected by
astronomers all over the world to examine the surroundings
of the Black Hole suspected to be present at the center of
our Milky Way galaxy. A variety of scientific instruments,
using different methods and different wavelengths have
been utilized to get many orders of magnitude of image precision.
This permits us to make a continuous fly-in starting from the familiar
constellations and going all the way in
to the stars actually orbiting the Black Hole itself.
|

|
The Sun's interaction with its environment.
This image of the heliosphere, representing the interaction
of the Solar wind with the surrounding interstellar material,
is taken from our short film "Solar Journey;" an extended
version of the film will be produced for public distribution on
videotape and DVD during the coming year. The
shapes depicted here utilize a theoretical model by Timur Linde from
the University of Chicago. The image has appeared as the Astronomy Picture
of the Day,
APOD 2002 June 24,
and was used as an illustration in a recent astronomy
news article in Science Magazine, page 2005 of
Vol. 300, 27 June 2003. (The image credit is very obscure, in tiny
vertically-aligned print along the spine of page 2005.)
|

|
Satellites in our Sky (GMT June 24th 2003 2:21pm).
We have over a thousand satellites flying through the sky over our
heads. This image is from a
brief animation representing a user's interaction with
our Earthday graphics program. The animation
shows a large portion of these at a
selected time, and then zooms in for a closeup of the International
Space station (ISS).
We can clearly see the ring structure of geo-stationary (deep-space) satellites
rotating with the Earth, located 38,500km
above the Earth's surface (about 6 times the radius of the Earth).
The entire animation appeared as the Astronomy Picture
of the Day on 14 July 2003. See
APOD 2003 July 14.
|
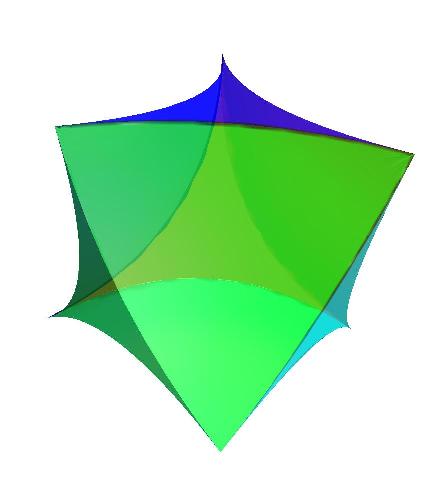
Calabi-Yau Cross Sections:
I have also created a variety of graphics images derived from the Fermat
Equation (see below) that are relevant to the Calabi-Yau spaces that may
lie at the smallest scales of the unseen dimensions in String Theory; these
have appeared in Brian Greene's books, The Elegant Universe
and The Fabric of the Cosmos,, and
in the book by Callender and Huggins, Physics
Meets Philosophy at the Planck Scale. The writhing purple shapes
in the October/ November 2003 NOVA production Elegant Universe,
as well as the cover of the November 2003 Scientific American,
were derived from software models I supplied to the NOVA graphics
providers.


These images show equivalent renderings of a 2D cross-section of the
6D manifold embedded in CP4 described in string theory calculations by the
homogeneous equation in five complex variables:
z05 + z15 +
z25 + z35 +
z45 = 0
The surface is computed by assuming that some pair of complex inhomogenous
variables, say z3/z0 and z4/z0, are constant (thus defining
a 2-manifold slice of the 6-manifold), normalizing the resulting inhomogeneous
equations a second time, and plotting the solutions to
z15 + z25 = 1
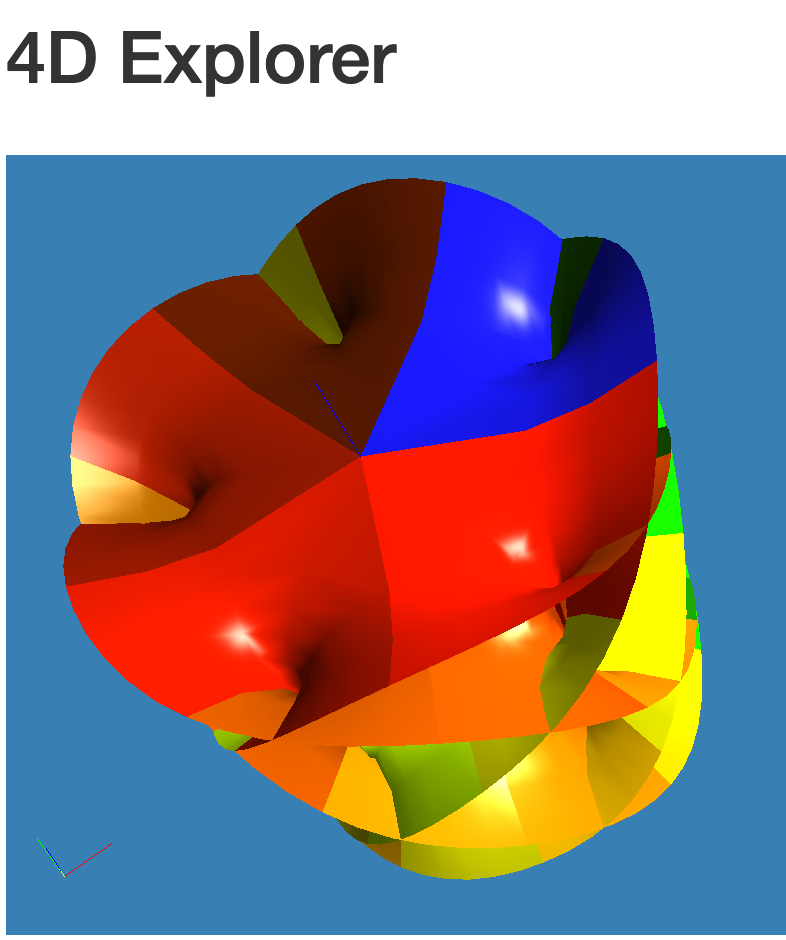
The resulting surface is embedded in 4D and projected to 3D using Mathematica
(left image) and our own interactive MeshView 4D viewer (right image). If
you have
CosmoPlayer, you can also
interact with this VRML version
of the quintic Calabi-Yau cross-section. This method is now obsolete,
as the WebGL
"4D Explorer" App runs on more platforms and has
a complete 4D interactive interface.
In the right-hand image, each point on the surface where five different-colored
patches come together is a fixed point of a complex phase transformation;
the colors are weighted by the amount of the phase displacement in z1 (red)
and in z2 (green) from the fundamental domain, which is drawn in blue and
is partially visible in the background. Thus the fact that there are five
regions fanning out from each fixed point clearly emphasizes the quintic
nature of this surface.
For further information, see: A.J. Hanson.
A construction for
computer visualization of certain complex curves.
Notices of the Amer. Math. Soc., 41(9):1156-1163, November/December
1994.
An interactive version is available at
the Wolfram Demonstrations Project Calabi-Yau
Space page, based on the Hanson paper cited above, with
assistance from Jeff Bryant. Most features of the latter
are now available on the WebGL
"4D Explorer" App, implemented by Leif
Christiansen, which runs on more
platforms and has a complete 4D interactive interface.
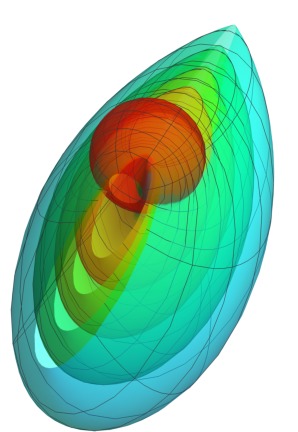
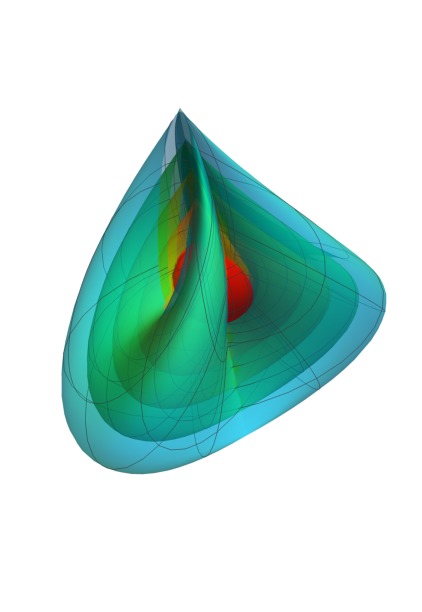
Arbitrary Genus Surfaces:
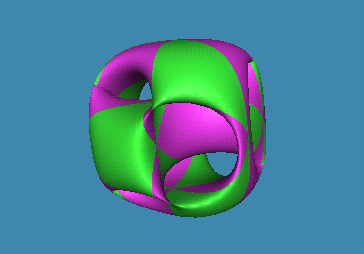 This image shows my computer graphics construction of a four-hole
torus described by an equation in complex two-space given by H. Blaine
Lawson, "Complete Minimal Surfaces in S3," Ann. of Math. 92,
pp.~335--374 (1970), with m = n = 2,
This image shows my computer graphics construction of a four-hole
torus described by an equation in complex two-space given by H. Blaine
Lawson, "Complete Minimal Surfaces in S3," Ann. of Math. 92,
pp.~335--374 (1970), with m = n = 2,
Im z1(m + 1) + | z2 |(m-n) Im z2(n+1)
= 0
and
|z1|2 + |z2|2 = 1
In general,
the genus is m*n, and this surface is not actually minimal in S3
except for
m = n = 0 and m = n = 1.
Review article
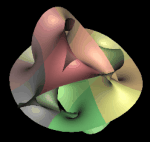 Cover picture: IEEE Computer 27 (July 1994)
Cover picture: IEEE Computer 27 (July 1994)
- For more information about mathematical visualization in general,
see our
review article (DOI:10.1109/2.299415):
Interactive Methods for Visualizable Geometry, by A.J. Hanson, T.
Munzner, and G. Francis, published in IEEE Computer 27 ,
No. 7, pp. 73--83 (IEEE Computer Society Press, Los Alamitos, CA, July,
1994). An online version is
here, and a local copy can be
found here.
Mathematics and Physics Animations
We have produced a number of short video animations with mathematical and
physical content. Some of my favorite projects are the following:
- Cosmic Clock:

Observing the Universe using the finite speed of light to place measured
objects in their correct temporal context.
- The Cosmic
Bloom excerpt from the movie as available here; it seems to play OK on
PC's with QuickTime, but has troubles on some other platforms.
- This 3:35 minute animation contains a visualization of the entire
Universe from three different points of view: the time spectrum of observable
photon radiation arriving at the earth, the constant-time shells of light
sources represented in "comoving coordinates " (as though the Universe
had always been the size it is today), and in "physical coordinates" (which
incorporate the Hubble expansion since the "Great Flash", when the Universe
was about 300,000 years old). This film was one of a select few chosen for
showing at the Siggraph 2000 Electronic Theater in July 2000, and appears
in Siggraph Video Review 134, Scene 5 (2000).
- Black Hole Flyin:

Approach to the Black Hole at the Galactic Core.
-
This animation represents an assembly of data collected by
astronomers all over the world to examine the surroundings
of the Black Hole suspected to be present at the center of
our Milky Way galaxy. A variety of scientific instruments,
using different methods and different wavelengths have
been utilized to get many orders of magnitude of image precision.
This permits us to make a continuous fly-in starting from the familiar
constellations and going all the way in
to the stars actually orbiting the Black Hole itself.
-
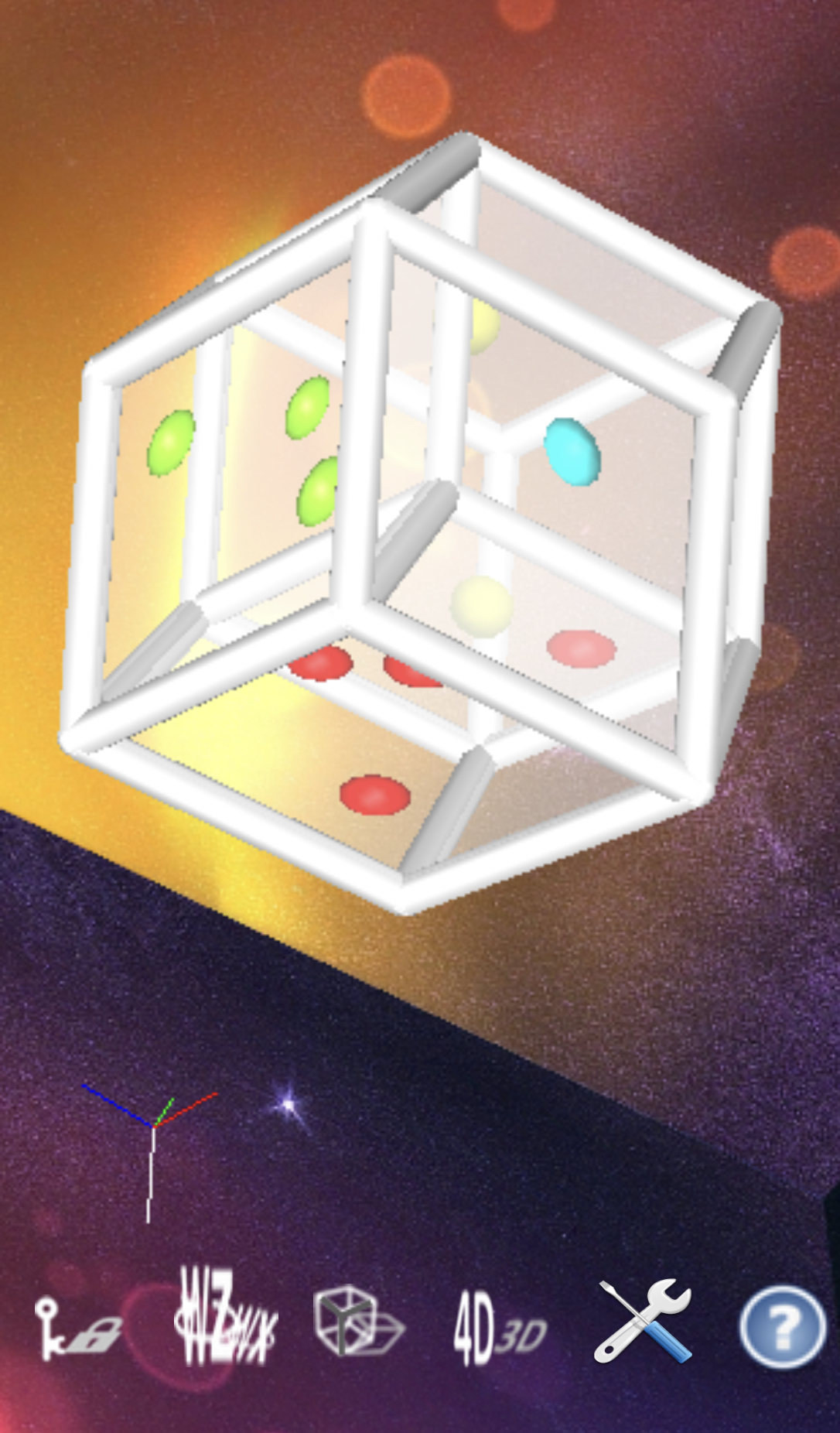
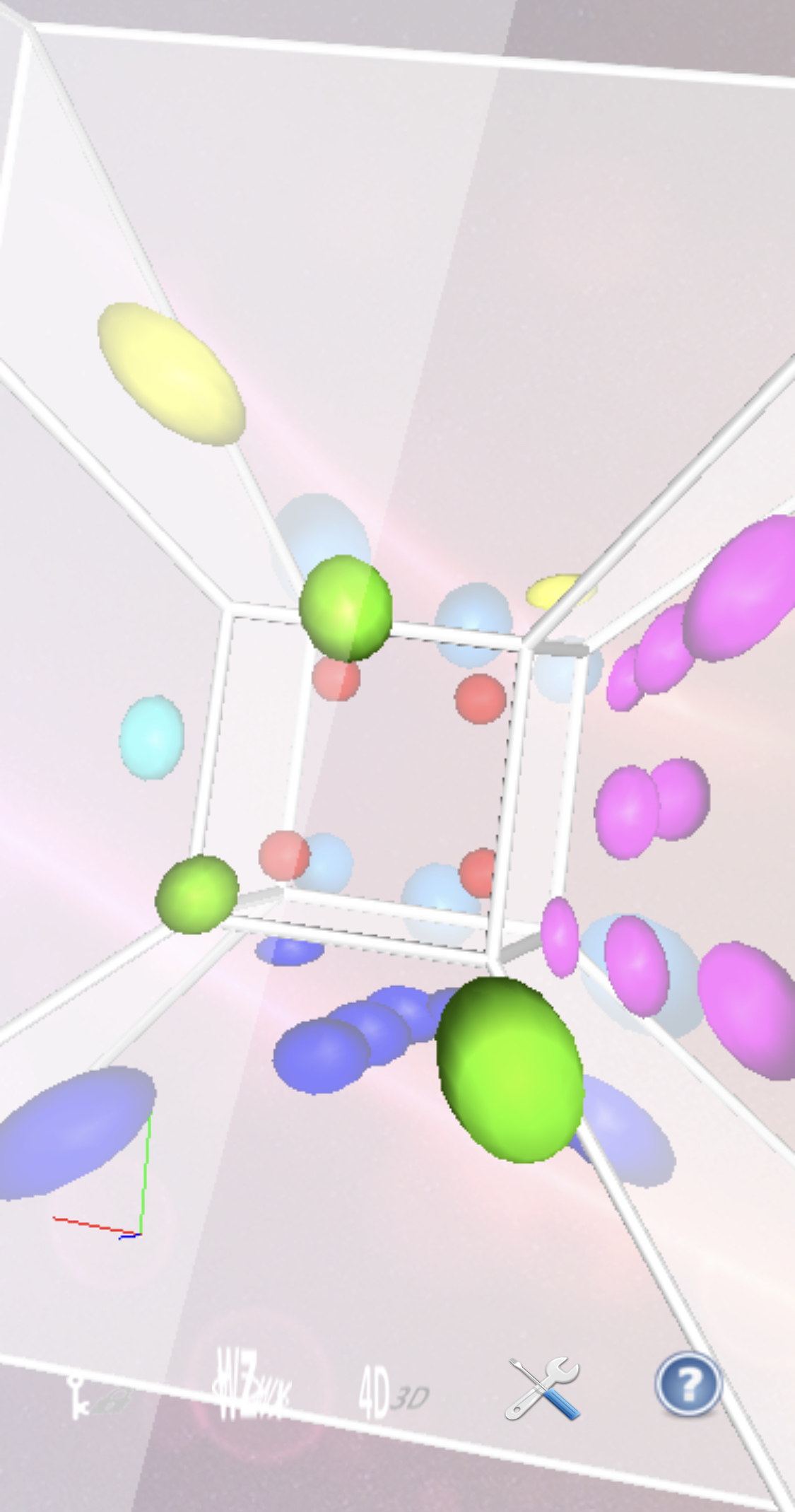
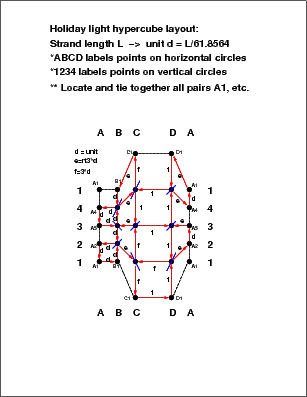
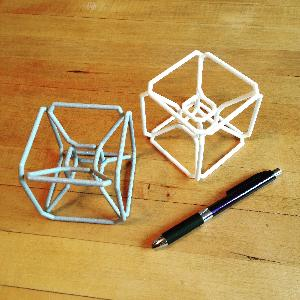
4Dice: Hypercube
 4Dice: A Glimpse into the 4th Dimension (MPG silent version)
4Dice: A Glimpse into the 4th Dimension (MPG silent version)
-
4Dice: Local version with narration.
-
4Dice: YouTube version
with narration.
- This short (1:00 minute) animation of a back-face-culled 4D die or
hypercube, which has eight sphere-containing cubes as the analogs of faces,
was shown at the Siggraph '95 Computer Animation Festival and appears in
Siggraph Video Review 114, Scene 14 (1995).
- Visualizing Fermat's Last Theorem:
 Visualizing Fermat's Last Theorem Video
Visualizing Fermat's Last Theorem Video
-
Fermat Video YouTube version:
- Fermat's last theorem was proven at last in 1995 by Andrew Wiles, but
the mystique lives on. This film was made in 1990, when it was still unclear
that Fermat's conjecture would ever actually be proven --- in a way it was
more romantic when we could believe that, wasn't it?
- The film was shown in the Siggraph '90 Animation Screening Room,
and appears in Siggraph Video Review 61, Scene 4 (1990).
Andrew J. Hanson: Last revised 12 December 2020.





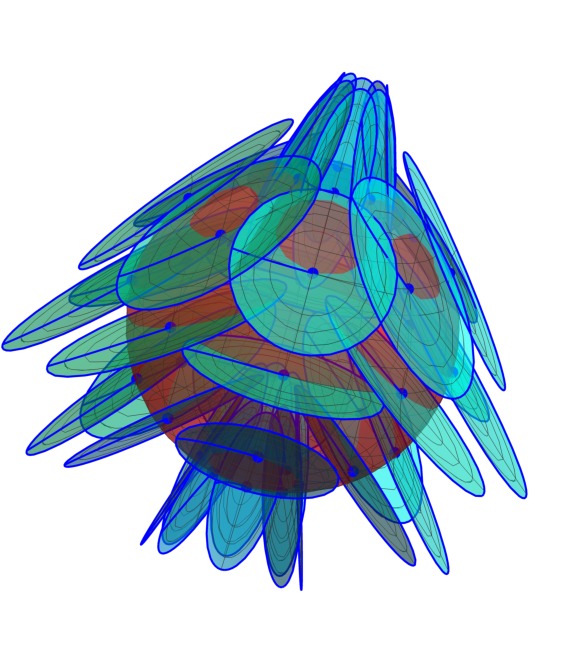
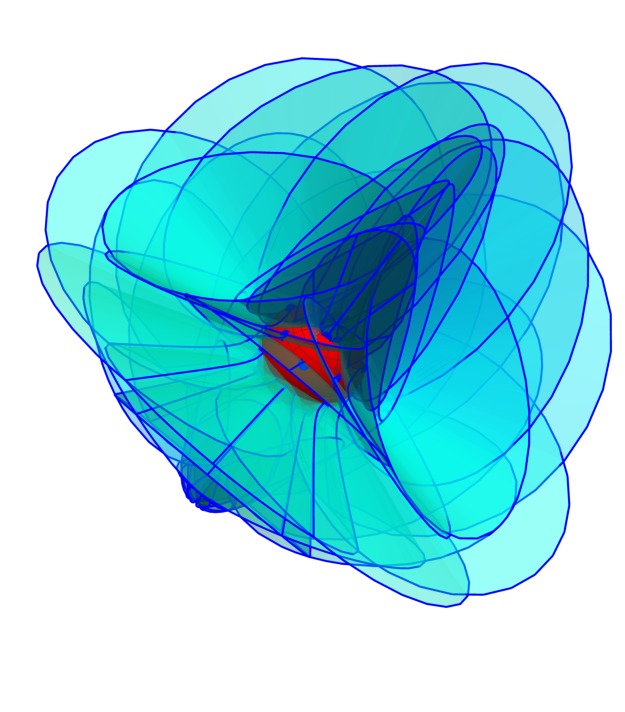





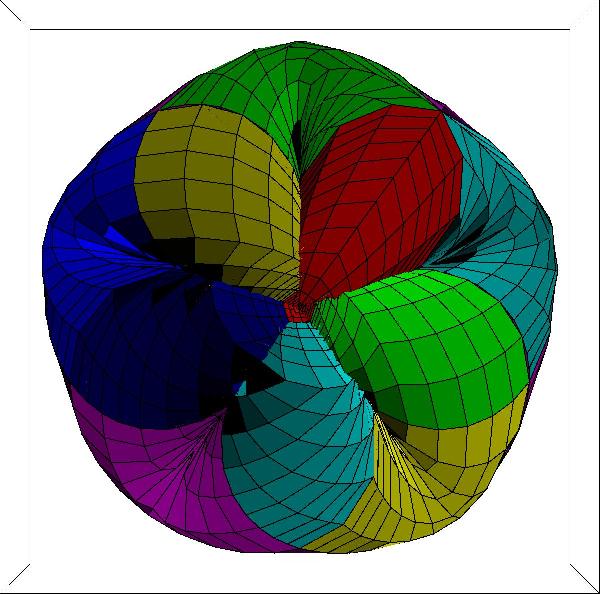





 This image shows my computer graphics construction of a four-hole
torus described by an equation in complex two-space given by H. Blaine
Lawson, "Complete Minimal Surfaces in S3," Ann. of Math. 92,
pp.~335--374 (1970), with m = n = 2,
This image shows my computer graphics construction of a four-hole
torus described by an equation in complex two-space given by H. Blaine
Lawson, "Complete Minimal Surfaces in S3," Ann. of Math. 92,
pp.~335--374 (1970), with m = n = 2,  Cover picture: IEEE Computer 27 (July 1994)
Cover picture: IEEE Computer 27 (July 1994) 